Retrotech — With a Future?

by

Before there were electronic digital computers, there were mechanical analog computers. Although now obsolete for practical computation, these devices might actually have an useful future ahead of them–in education.

Mechanical analog computation (analog means that calculation is done by measuring rather than by counting) goes back to the Greek Antikythera mechanism (65 BC), which was used to predict the positions of heavenly bodies. The modern era of analog computing began with the work done by James Thompson and his brother William (Lord Kelvin) in the 1870s. First, James Thompson created a mechanical device that performs the calculus function of integration.

Lord Kelvin applied this device…along with other mechanisms for addition and trigonometric functions…to create a mechanical tide-prediction system. These tide predictors had a pretty good run: the invention was announced in 1876, and some of these systems were still in use in the early 1970s!

For those who haven’t studied calculus, integration can be thought of as a kind of continuous addition.  Imagine a hose with a fluctuating flow rate filling a pool: by integrating the rate of flow, you can calculate the volume of water added to the pool.

The basic concept of a mechanical integrator is shown below.

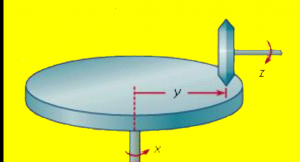
mechanical-integrator-modified

If the vertical shaft is turned at a constant rate, and the small wheel is moved in and out according to the changing value of some some variable Y, then the rotation of the horizontal shaft Z will represent the integral of Y with respect to time.  If Y is the rate of flow of the a hose, Z will be the total volume  added to the pool. If Y represents the acceleration of a vehicle, then the output shaft will give that vehicle’s speed at any moment.  Connect the output to the input of another integrator, and you will get the distance traveled.

Vannevar Bush, who would become Roosevelt’s science adviser during WWII, combined the integrator and other computing mechanisms to create a highly general mechanical computer, called a differential analyzer. Completed in 1931, it was not restricted to a single application, but could be programmed–with a wrench and screwdriver to alter the connections–for a wide range of problems. Complex chains of calculation were possible, including the ability for a result at one stage to be fed back as input at an earlier stage–for example, the speed of a simulated vehicle affects its air resistance, which in turn influences its acceleration…which integrates back to its speed.

Other differential analyzers were built in the U.S.,  Norway, and Britain, and were used for applications including heat-flow analysis, electrical network stability analysis, soil-erosion studies, artillery firing table preparation, and studies of the loading and deflection of beams. It is rumored that a British analyzer was used in the planning for the bouncing-bomb attack on German hydroelectric dams during WWII. Differential analyzers appeared in several movies, including the 1951 film When Worlds Collide  (video clip). The ultimate in mechanical analog computation was the Rockefeller Differential Analyzer, a rather baroque (and very expensive) machine built in 1942. It was decommissioned in 1954, on the belief that the future of calculation would belong to the electronic computer, and especially the electronic digital computer.   Following the decommissioning, the mathematician Warren Weaver wrote:

It seems rather a pity not to have around such a place as MIT a really impressive Analogue computer; for there is vividness and directness of meaning of the electrical and mechanical processes involved… which can hardly fail, I would think, to have a very considerable educational value. A Digital Electronic computer is bound to be a somewhat abstract affair, in which the actual computational processes are fairly deeply submerged.

 

Vannevar Bush himself wrote about the machinist who had helped in construction of the original differential analyzer:

I never consciously taught this man any part of the subject of differential equations; but in building that machine, managing it, he learned what differential equations were himself … it was interesting to discuss the subject with him because he had learned the calculus in mechanical terms — a strange approach, and yet he understood it. That is, he did not understand it in any formal sense, he understood the fundamentals; he had it under his skin.

Not just integration, but other mathematical operations as well, have mechanical interpretations which could be useful for development of conceptual understanding.  Addition is done by a differential, which combines the rotation of two input shafts into the rotation of a common output shaft.  A mathematical function can be mechanized with a cam…math textbooks sometimes try to explain the concept of a function in terms of an abstract “function machine”….with the differential analyzer, the abstraction becomes concrete.  Mechanical multiplication, too, can be provided and is useful in developing conceptual understanding.

In 2004, Marshall University professors Bonita Lawrence and Clayton Brooks visited the London Science Museum and saw a differential analyzer on static display.  Dr Lawrence was impressed by the educational possibilities of such a device, and created a project to begin exploring them.  The team located Dr Arthur Porter, who had created the differential analyzer at Manchester University and later moved to the United States; they were further inspired by the resulting discussions.  They also saw a working differential analyzer constructed in California by Tim Robinson:  “The magnitude of the task of building such a machine of our own literally came to life as we watched the wheels and gears turn according to the mathematics that guided them.”

The two professors and their associates created a student team and initially built a 2-integrator machine named Lizzie: see their paper, A Visual Interpretation of Dynamic Equations.  I read about the project and was intrigued enough to contact Dr Lawrence, who kindly invited me to come out and visit: I did, and was impressed with the work being done and the spirit of the students involved. At the time they were just beginning work on a 4-integrator machine, which is now complete and has been named Art, in honor of Dr Porter.  Some photographs.

(Good grief–has it been 11 years already?)

I was reminded of this work by a post at The Lexicans, in which Lex remarked that he had not done terribly well at math in high school and the first two years of college:

It was not until my junior year at the Naval Academy, when we started to do differential equations, that the light came on. Eureka! Drop a wrench from orbit, and over time it would accelerate at a determinable pace, up until the moment when it entered the atmosphere, where friction would impede the rate of acceleration at an increasingly greater rate (based on air density, interpolated over a changing altitude) and that wrench struck someone’s head at a certain velocity, that any of this applied in the real word. By then it was too late, I was too far gone, and an opportunity was lost.

I think the use of a mechanical differential analyzer would be of great assistance to many students in helping the light to come on, and also I think it would be of value in giving some understanding of differential equations and mathematical modeling to a large number of people who will never be exposed to the formal derivations studied in a normal calculus class but could still benefit from a little intuitive grasp of what it’s all about.

I hope the Marshall group is being successful in interesting high schools and other universities in the potential of these analyzers.  My guess is that it’s somewhat of an uphill battle given the too-common preferences of the educational establishment for what Michael Schrage called ‘sparkly tools.’  Of course, it would not be difficult to create a simulation of a mechanical differential analyzer that runs on a regular desktop or laptop computer, with visual effects for spinning wheels and shafts, etc…this might add ‘sparkle’, but I think it would loose much of the value.  From an article in Capacity magazine:

 “What’s great about this is that students can ‘see’ different equations and the impact on variables, which represent the rates of change of the solution you are interested in,” Lawrence said. “It isn’t the same with computer simulations,” she added. Here, screwdrivers are useful.

I think there are probably a significant number of people who will learn in an environment where ‘screwdrivers are useful’ better than in a wholly digital environment, even one which is simulating an analog approach.

27 thoughts on “Retrotech — With a Future?”

  1. There were electronic analog computers also. My father worked on one that modeled the electric utility’s system he worked for. They were also used to simulate river systems. The Corps of Engineers had a big one for the Mississippi river system. This was in the ’50’s, he was also working to develop digital simulations at the same time.

    Less elaborate mechanical computers were fairly common. There was a post here a few years ago about the computers used to direct the guns on an ship. The Norden bomb sight was another. They were a bear to keep working.
    https://www.youtube.com/watch?v=s1i-dnAH9Y4

    Babbage’s difference engine was based on a completely different principal.
    https://www.youtube.com/watch?v=BlbQsKpq3Ak

    If anyone feels up to producing a computer simulation, I would suggest they look into a program called blender.
    https://www.blender.org/

    Some might find it helpful in learning calculus, but my experience is that there isn’t so much a shortage of people that understand the math as too few that can make the connection between math and the real world. And too few of those that can are willing to work for cheap. Still, the more the merrier.

  2. MCS…”my experience is that there isn’t so much a shortage of people that understand the math as too few that can make the connection between math and the real world”

    Agree…and I think it is precisely this lack that creative teaching tools like the differential analyzer can help with, both among the people that are going to be doing the modeling work and those that are sponsoring the work and looking for the results.

  4. I took the last class in analog computers offered in my engineering school circa 1976. They were the electronic sort and we even had a lab.

    I also took a NROTC class (to avoid PE) on weapon systems and we had a destroyer man as an intructor. He was wildly impressed with the gun fire controller on his ships.

    I even went back and got a Kindle eBook on the development of naval fire controllers that had a bit TMI for me.

    https://www.amazon.com/gp/product/B00KTI0T0E/ref=ppx_yo_dt_b_d_asin_title_o08?ie=UTF8&psc=1

  5. When I worked at Douglas Aircraft in 1959, I was programming an IBM 650 while my girlfriend at the time was programming and operating an analog computer in the main plant. I was in the wind tunnel facility.

    “Programming” is a bit of a stretch as the machine only had 2000 addressable memory spaces on a rotating drum. The memory was for the program and the data was all on punched cards.

  6. I’m trying to grasp it:

    If the vertical shaft is turned at a constant rate, and the small wheel is moved in and out according to the changing value of some some variable Y…

    I only see one wheel. Are you saying that the entire wheel/shaft assembly moves closer to / further from the “record player needle”?

    My calculus teacher was just off the boat from Singapore where apparently students work their asses off and never question the teacher about anything. I went into the class hoping to understand how Excel’s “Solver” worked because I used it when marketing would give me their sales forecast and I had to optimize the factory’s production line. I had no problem figuring out how to do that in Excel and had been doing it for years before the class. I quickly learned how to do it on my TI-89 that we were allowed to use in class. But we had to show our work. I could easily do a simple differential equation by hand but I thought it was a waste of time doing complex equations like that when we had calculators. The professor and I had quite a few discussions about that. He was a good guy but wouldn’t budge from his dogmatic ways. I got a B in his class.

  7. CR….the large horizontal wheel (disk) is turned at the constant rate. It is the small wheel that moves in and out, via a linkage driven by some shaft. When the value of the variable being integrated is zero, the small wheel is at the center, so it doesn’t turn. When the value is at its maximum, then the small wheel moves to the outer edge and spins fast, thereby increasing the result variable rapidly.

    This is for integration with respect to time: it is also possible to drive the large wheel/disk via another variable and thus to integrate with respect to that variable.

  8. My calculus teacher was just off the boat from Singapore where apparently students work their asses off

    My Calculus teacher was a tiny little Indian with a thick accent. This was 1957 and I was a scholarship student. My work habits were still undeveloped and I had not done much of the homework. I needed a B in the class to keep my scholarship. I talk to Mr Bashwa and was told I would get a B if I got an A on the final exam. I settled down, finally, and got the A. When grades were posted, I had gotten a C in the class. I made an appointment to meet him and he did not show up. I made another with the same result. I was walking down University Avenue a few days later when I saw him across the street. I called to him and began to cross. He saw me and began to run away. I decided chasing him was probably not going to change my grade.

    I worked at Douglas for a year, then went went back to night school and switched to premed.

  10. “What’s great about this is that students can ‘see’ different equations and the impact on variables, which represent the rates of change of the solution you are interested in,”

    That’s often the key with math, grasping what’s going on under the hood by relating it to real world empirical experience. That’s the big advantage of graphs, charts, and other visualizations and we see a lot of that modeling nowadays.

    Although, for other types of math that isn’t going to be the case. Similar to philosophy, there’s a difference between the synthetic statements of applied math vs the analytic statements of mathematical logic and proofs. In the case of the latter, good old-fashioned intuition is the way to get a feel for it. Not so easily taught, if it can be taught at all.

  11. I can’t help but think that a lot of the problem in math teaching is calculators, especially in elementary school. I know that I never would have learned the multiplication tables and long division if I’d had one. I used a slide rule from the 6th grade but that’s a completely different animal. I think you miss getting a feel for numbers if all you ever see are carried out to 10 significant figures. With a slide rule, you had better have an idea of at least the order of magnitude of your answer or it will come back to bite you. I’ve gotten as lazy and out of practice doing mental math as anyone, but I can remember being better.

  12. MCS…also, I can’t imagine conducting a financial negotiation session very well without having some kind of feel for numbers. Sure, you can have your calculator with you, but the number-literate person has a huge advantage over the person lacking such literacy.

  13. MCS–you are absolutely right. As a veteran of the schools, I can tell ya — I was in sped, and at least there, we were responsible for curriculum. But the problem was ALWAYS other teachers, dept heads, etc handing my kids all kinds of little devices (this was before everyone had a laptop)…or at least they’d want them to use written tables.

    Could not get them to understand that by learning foundational content, it opened new, neural pathways that made other learning easier and it was info that could be generalized for other situations.And too many parents would want kids in those classes– “0h, she knows so much,” they’d say, just because the teacher used a lot of silly jargon and technology in place of learning.

    Same even with handwriting–the others would say “give them a device,” where I’d be having them practice the skill itself. But anything traditional is scorned, and they esp hate “drill and kill,” as they call any memorization.

    However, my sped kids could find all kinds of countries and cities on a map or globe, where the reg ed kids could not, as well as being able to write (they don’t even teach cursive anymore) and memorize times tables (they’d get rewards for each new one memorized.) Left schools almost a decade ago, but my husband is still there, and education hasn’t changed since it became “fads over content” in the 70s-80s.

  15. I dispute the value of cursive. I have never seen evidence it improves anything.

    To the main point: According to James Gleik’s “Chaos,” Doyne Farmer and Norman Packard logged hours on an analog computer and credited this with a deeper understanding of complex systems

  16. Cursive solved a big problem – quill pens didn’t like stopping, starting, or changing writing speeds much. Making block letters with quill pens was very hard. So a whole new style of writing was developed to accomodate their shortcomings.

    We don’t use quill pens any more, and there’s no reason for cursive to exist.

    I had to learn to write cursive in elementary school. We never had to read it, so I never learned how, not past puzzling over each letter.

    I rebelled against the whole thing before I got to junior high, when I realized if cursive was so wonderful, why were all of my schoolbooks printed in block letters? Why did I never see cursive outside of the classroom, other than logos and advertising? Other than my signature, I haven’t used cursive since.

  17. I rebelled against the whole thing before I got to junior high, when I realized if cursive was so wonderful, why were all of my schoolbooks printed in block letters? Why did I never see cursive outside of the classroom, other than logos and advertising? Other than my signature, I haven’t used cursive since.

    Well, interestingly, I found my cursive getting unreadable. So I switched to block printing. And as THAT sped up, I could see where cursive came from, as some of my block printing began to resemble cursive.

  18. Two things:

    1) Vannevar Bush is the author of As We May Think
    https://www.theatlantic.com/magazine/archive/1945/07/as-we-may-think/303881/
    “Consider a future device … in which an individual stores all his books, records, and communications, and which is mechanized so that it may be consulted with exceeding speed and flexibility. It is an enlarged intimate supplement to his memory.”

    Published in 1945.

    It describes the internet.
    He got the technology totally wrong. But his device is remarkably close to a description of the internet.

    It’s a fairly long piece, but well worth the read.

    2) Analog Computers. They could be the source of the answer to Asimov’s Last Question (1956)
    https://en.wikipedia.org/wiki/The_Last_Question
    It’s long been my favorite Asimov, and it was apparently his own favorite, too.
    Yeah, I know. “Automatic Computer”. But it’s an amusing idea…

  19. “What’s great about this is that students can ‘see’ different equations and the impact on variables, which represent the rates of change of the solution you are interested in,” Lawrence said. “It isn’t the same with computer simulations,” she added. Here, screwdrivers are useful.

    I do not see this at all. Students today more than amply grasp the idea of changing perspectives, and of simulating real things using a computer.

    I have zero doubt that the sort of mind which needs/uses this kind of help§ can and would be able to see wheels and dials in a simulated AC as the same as real world wheels and dials. It’s the kind of mental substitution which kids today are very much trained in from an early age: seeing the computer model as the real world thing (it also leaves them vulnerable to believing the model is correct when it has broken down, but that’s a different issue entirely)

    ===
    § I say “needs/uses” because there are certainly people who don’t, me being one of them. I have zero doubt that, had those useless idiots teaching me math been using a pipeline rather than a teaspoon, that I could easily have been doing calculus by age 12. As it was, I set up to take it as a 10th year Junior in high school (skipped a grade) @ 15, and the idiots would not let me, which pissed me off enough that I went ahead and checked out a college textbook and taught it to myself… I have always soaked up math like a sponge, it comes quite naturally to me. That’s not a superiority thing, there are other skills that don’t come naturally, but I was taking grad level math (5000) courses as a sophomore in college, because I had the prereqs, they did not stop me, and the teacher was one of the best I’ve ever had. I did not need anything like this to gain comprehension. But other minds learn differently, or at perhaps “better differently” might be a more suitable term.

  20. TRX:

    I rebelled against the whole thing before I got to junior high, when I realized if cursive was so wonderful, why were all of my schoolbooks printed in block letters? Why did I never see cursive outside of the classroom, other than logos and advertising? Other than my signature, I haven’t used cursive since.

    After a bit of development, cursive writing is faster than block printing. Your textbooks and other works were printed in block print because it’s much easier to work with fonts that are not cursive (at least until the computer age).

  21. OBH…”Students today more than amply grasp the idea of changing perspectives, and of simulating real things using a computer.”

    I would say, rather, than students today understand that people simulate real things using a computer, and many of them have actually run such simulations themselves. Understanding how such simulations are actually done, and how they model the real world, and what the strengths and weaknesses of these simulations might be, is another matter.

  22. I think what the discussion above illustrates is the different people learn the same concepts in different ways. I tend to approach problems numerically, The appeal of graphing calculators has always been lost on me. I love digital displays to the point that I have to think for a second when confronted with an analog clock. I know there are many others on the other side. It’s not a question of better it just seems to be a difference between people like hair color.

    What this tells me is that there is never going to be a single best way to teach anything. I’ve spent many hours pushing and prodding at some problem or concept without making any headway until I happen upon a thought or statement somewhere and it all falls into place. I can remember, what seems now a very short period, where reading went from being a chore to reading everything that I could get my hands on, often to the displeasure of teachers.

    I think that I am decent at teaching one-on-one or a very few. This allows me to see easily when I am talking past my student and that I need to find a different approach. Otherwise, I’m just pounding away at my square peg annoying both of us. The few times that I have been in a one-to-many situation has lead to two conclusions. First: It’s not my strong point, not surprisingly, since possible lack of talent aside, the skill takes practice. Second: Those that are good at it deserve respect for mastering a difficult skill. This probably explains a lot of the success that home schooled students seem to have. The teacher is naturally motivated to motivate ALL of the students.

    The trivial corollary is that classroom teaching is hard to do well. Unfortunately, only a fraction of the people we pay to do it are even passable at it. The future of a lot of kids seems to depend on having at least one of the exceptions during their education and it’s a crap shoot.

  23. I dispute the value of cursive. I have never seen evidence it improves anything.

    AVI,

    I was the Scoutmaster of the local troop for over 18 years. Back in 2005 I noticed that the new scouts we got in were having a LOT of trouble learning to tie knots and use a knife without cutting themselves. Out of 7 newbies, only one was not having trouble. A couple of months later I had all the boys sign a “thank-you” card to a large donor. All of the newbies except the one who was not clumsy fingered signed their names with big block letters like a 1st grade kid. When I asked them if they knew how to write cursive, they all looked at me with puzzled looks. Later I asked one of the moms who was an elementary school teacher about it and she told me the school dropped teaching cursive 3 years earlier. Over the next two years I noticed more kids with very little fine motor skills in their fingers, except for those who went to private schools or were home schooled.

    Then it hit me, the act of learning cursive developed fine motor skills in boys with the constant repetitive practice. IMHO, it IS NOT a waste of time, but as someone else earlier noted, modern teachers are indoctrinated with hatred of the “drill and kill” education methods, which worked very well, but caused the teachers to do more work also. I started passing on this little tidbit to others here, and lo and behold, 5 years ago, the local elementary schools brought back teaching cursive, though not as intensively as before, but enough that most of the kids we got before I stepped down as Scoutmaster had better fine motor skills and did not have as much trouble.

  24. MCS….”I think what the discussion above illustrates is the different people learn the same concepts in different ways.”

    The Neptunus Lex post offers a good example of this. Lex, obviously a brilliant man, said that math didn’t really come together for him until he was introduced to the dynamic world of differential equations and their connection to the world of moving physical objects.

  25. @David Fostger:It is the small wheel that moves in and out…

    Doing an image search using that image’s URL, I realize my error: I was seeing the small wheel as a needle, not as a wheel on end. Joe Biden’s suggested child method must be stuck in my head.

Comments are closed.